About

1.5 Example of investigate the motion of an oscillator using experimental and graphical methods

1.5.1.Q1: what is the maximum angle of release before the motion is not accurately described as a simple harmonic motion for the case of a simple free pendulum?

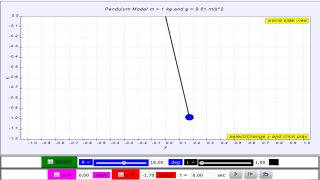
Example 1: Simple pendulum A pendulum bob given an initial horizontal displacement and released to swing freely to produce to and fro motion

1.5.2 Suggested Inquiry Steps:

1.     Define the question in your own words
2.     Plan an investigation to explore angle of release to record the actual period T and theoretical period  ${\displaystyle T_{the\text{or}y}=2\pi \sqrt{\frac{L}{g}}}$  where L is the length of the mass pendulum of mass, m and g is the gravitational acceleration of which the mass is experiencing, on Earth's surface  g = 9.81 m/s²
3.     A suggested record of the results could look like this   

angle / degree	T /s	Ttheory / s	${\displaystyle err\text{or}=\frac{(T-T_{the\text{or}y})}{T}100\%}$
05
10
15
20
30
40
50
60
70
80
90

 
        With the evidences collected or otherwise, suggests what the conditions of which the angle of oscillation can the actual period T be approximated to theoretical period such that  T  ≈ ${\displaystyle T_{the\text{or}y}=2\pi \sqrt{\frac{L}{g}}}$

1.5.3 Suggested Answer 1:

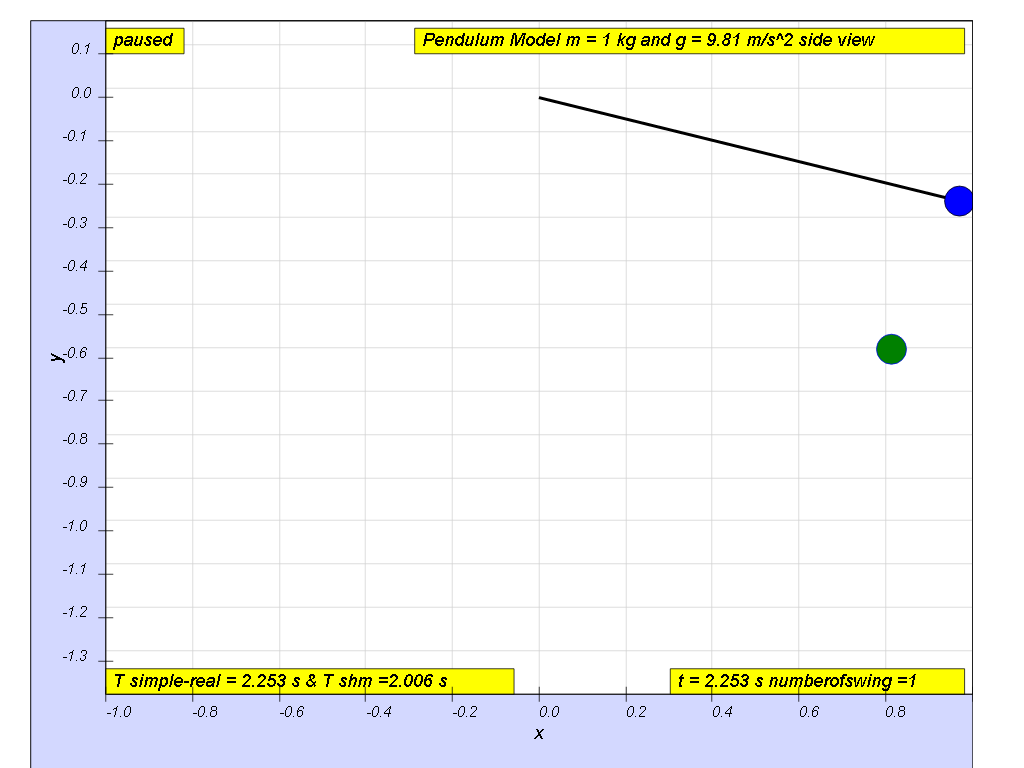
 angle θ  ≈ 10 degrees for ${\displaystyle err\text{or}=\frac{(2.010-2.006)}{2.010}\left(100\right)=0.2\%}$, depending on what is the error acceptable, small angle is typically about less than 10 degree of swing from the vertical.

1.5.4 Conclusion:

Motion approximates simple harmonic motion when the angle of oscillation is small < 10 degrees..

1.5.5 Other Interesting fact(s):

Motion approximates SHM when the spring does not exceed limit of proportionality during oscillations.

1.5.6 Real Life Application of Small Angle Approximations:

Astronomical applications of the Small Angle Approximation

1.5.7 YouTube

http://youtu.be/BRbCW2MsL94?t=2m16s This video shows many pendulums that goes in phase and out of phase with each other pendulum to creating a visually stunning effect.

1.5.8 Model:

1. Run Sim
2. http://iwant2study.org/ospsg/index.php/93

 

Translations

Code	Language		Translator	Run

Credits

This email address is being protected from spambots. You need JavaScript enabled to view it.

http://iwant2study.org/lookangejss/02_newtonianmechanics_8oscillations/ejss_model_SHM195/SHM195_Simulation.xhtml

 Apps

https://play.google.com/store/apps/details?id=com.ionicframework.shm195app415781&hl=en

end faq

{accordionfaq faqid=accordion4 faqclass="lightnessfaq defaulticon headerbackground headerborder contentbackground contentborder round5"}