updated 20250016
-
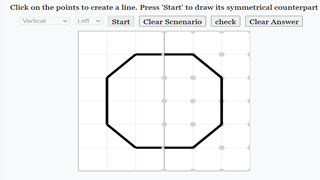
xAPI Exploring Symmetry with the Shaded Symmetry Simulation by meiYoke
xAPI Exploring Interactive Nets of Cube and 3D Visualization with the New EJS Simulation 🌟🔦🎨 by meiyoke
xAPI Exploring the “Thinking Aloud Road” Simulation for Primary Math by meiYoke
xAPI Exploring Fractions with xAPI-Enabled Interactive Learning by meiYoke
xAPI Exploring Line Symmetry with WebEJS: A Hands-On Interactive by meiyoke
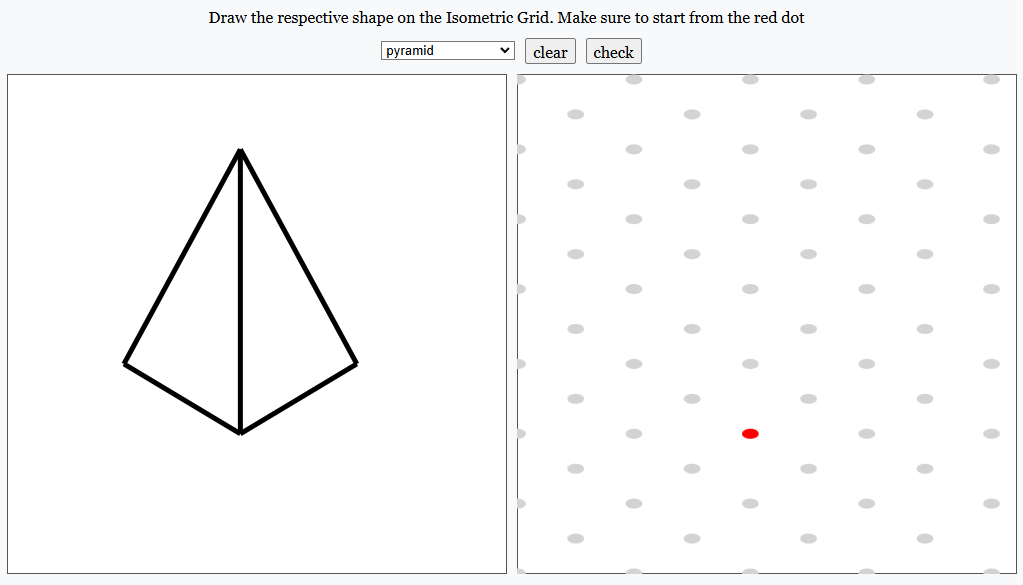
xAPI Drawing 3D Shapes on an Isometric Grid: A Fun and Educational Tool by meiyoke
xAPI Thinking Aloud: Fill the Tank – Interactive Math Puzzle by meiyoke
xAPI Interactive Math Learning: Estimation Made Fun with EJS by MeiYoke
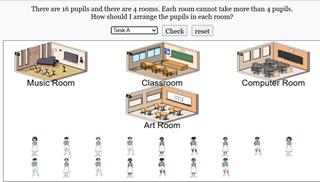
xAPI Engaging Students with Interactive Learning: An EJS Simulation for Room Arrangement Challenges by MeiYoke
Artifacts
Credits
['kangRui', 'meiYoke', 'looKang']
https://sg.iwant2study.org/ospsg/index.php/interactive-resources/mathematics/1270-math-estimation-v3
Credits
['kangRui', 'meiYoke', 'looKang']
Credits
['kangRui', 'meiYoke', 'looKang']
https://sg.iwant2study.org/ospsg/index.php/interactive-resources/mathematics/378-isometric-grid-v4
Credits
['Kang Rui', 'meiYoke', 'looKang']
Credits
['Kang Rui', 'meiYoke', 'looKang']
 |
| demo PrimaryMathThinkingAloudRoadSimulation/ PrimaryMathThinkingAloudRoadSimulation.zip |
Credits
 ['lookang (This email address is being protected from spambots. You need JavaScript enabled to view it.)', 'sithu', 'idea by meiyoke']
['lookang (This email address is being protected from spambots. You need JavaScript enabled to view it.)', 'sithu', 'idea by meiyoke']
 |
webejs_model_Symmetry_Shaded_xAPI_v2.zip
webejs_model_Symmetry_Shaded_xAPI_v2/
Principal Investigator L M Yoke (Lead Specialist)
Co-Principal Investigator (Co-PI) Lawrence Wee Lead Specialist Technology for Learning/ETD/MOE
This email address is being protected from spambots. You need JavaScript enabled to view it.
Division / Branch
CPDD 1 /SCSB
Research Title
Use of Digital Simulation-based lessons to enhance Metacognition in Mathematics
Foundational Research Area / Futures Strand from General Education Research Agenda
Futures Strand: Future of Learning
Target Level
Primary
Student Profile
All Primary School Students
RESEARCH ABSTRACT (Provide a 200 words summary about your research proposal)
To develop students’ metacognition and mathematical processes and thinking (such as critical, adaptive, and inventive thinking - CAIT), the 2021 Primary Mathematics Curriculum textbooks series included thinking tasks that involve authentic real-world situations and allow for multiple solutions by exploring and discovering new ideas or different ways of working mathematically. These tasks can be expanded into more complex problems or simplified to suit different learning needs. Developing such tasks as digital simulations provide more opportunities for students to explore further. Students who need scaffolding can start with a simpler problem and gradually progress to more complex ones. These simulation-based thinking tasks offer a rich exploratory learning experience in a safe environment for diverse learners, students need not worry about how many attempts they made as compared to their peers. Since these tasks are based on the current textbook series, they are in alignment with the content, focus, and emphasis of the primary mathematics curriculum.
Therefore, we propose to develop open-source mathematics learning simulations based on thinking tasks in the primary mathematics textbooks. These simulations will be compatible with any modern mobile device and created in an efficient and sustainable manner. They will be made available on the Student Learning Space (SLS) for teachers to use in class or for students to engage in self-directed learning. Simulation-based lessons that promote CAIT and develop metacognition will be trialed and made available to teachers on SLS as resources.
RESEARCH OBJECTIVES (State the objectives of the research proposal clearly and succinctly in order of priority. Please indicate if this proposal is part of a larger study.)
To develop open-source mathematics learning simulations aligned to the Primary Mathematics curriculum;
To study the effectiveness of simulation-based lessons that enhance learning for diverse learners and foster thinking and metacognition in mathematics.
As a standalone, the proposal generates value through use of digital-based learning to develop metacognition and the 21CC of critical and adaptive thinking. This proposal could also be part of a larger study to explore Interactive Digital Textbooks (IDTs), including AI enabled features, should there be later plans to develop IDTs at the primary level. These learning simulations when put together with other resources such as AI-ALS and FA-Math could form part of the IDT that provides personalise learning experiences.
RESEARCH QUESTIONS (State the research questions that your proposal seeks to address.)
RQ1: How can teaching and learning practices be enhanced with the use of mathematics simulation to support students’ metacognitive growth?
RQ2: What are some ways in which teachers can improve in their teaching instructions so that students can benefit from learning through the mathematics simulations in enhancing metacognition in mathematics?
IMPETUS FOR RESEARCH (Provide a brief review of the literature or case for support of no more than 1000 words for your research proposal. Ensure that key constructs are defined. Append as a separate Annex if necessary)
The impetus for this research stems from the significant potential of simulations to enhance learning outcomes in education, particularly in the domain of mathematics. Simulations offer a dynamic and interactive platform that allows learners to engage with abstract concepts in a tangible and experiential manner. As highlighted by Ramdel et al. (1992), simulations provide learners with opportunities to actively participate in skill acquisition, thereby potentially facilitating deeper understanding and retention of knowledge.
One of the key advantages of simulations is their ability to mirror real-world situations in a simplified yet meaningful way. This enables learners to apply their knowledge and skills in authentic contexts, thereby bridging the gap between theory and practice. Moreover, simulations can be adapted to cater to learners of various ages and abilities, offering different levels of difficulty to accommodate individual learning needs. This adaptability allows students to progress from simpler tasks to more complex ones within the same conceptual framework designed in the simulation, promoting incremental learning and mastery.
In the realm of mathematics education, research has demonstrated the efficacy of simulation-based lessons in facilitating sense-making and conceptual understanding. By providing interactive digital simulations coupled with guided lesson activities, educators can create immersive learning experiences that actively engage students in the exploration and application of mathematical concepts. This approach not only fosters deeper conceptual understanding but also cultivates critical thinking, problem-solving skills, and metacognitive awareness among learners.
Thus, the impetus for this research lies in harnessing the potential of open-source mathematics simulations to transform teaching and learning practices in primary mathematics education. By distilling key design principles and measuring their impact on learning outcomes, this research seeks to provide empirical evidence supporting the effectiveness of simulation-based approaches in enhancing students' thinking skills, metacognition, and overall learning outcomes in mathematics. Through this exploration, educators can gain valuable insights into optimizing instructional practices and leveraging digital technologies to foster meaningful and engaging learning experiences for all students.
While there are existing lessons packages on the teaching of primary mathematics using technology, few leverage thinking tasks to develop students’ metacognition in mathematics. Building on these thinking tasks developed by the CPDD Mathematics Unit in the current primary mathematics textbook series, we propose developing simulations of these tasks to achieve greater blended learning with the primary mathematics curriculum. This development will guide teachers in integrating the best of both digital and physical manipulatives, using both traditional paper-and-pencil tasks and simulation-based lessons where appropriate. The simulations afford for self-directed learning on SLS. Teachers need to facilitate discussions among students and prompt them to think deeper whether it is a paper-pen task or a simulation. Teachers must develop the skill to engage students into thinking critically, inventively, adaptively after students have attempted the task individually. Critical, Adaptive and Inventive Thinking (CAIT) enables students to use sound reasoning and metacognitive skills to inform decision-making, generate novel and useful ideas to address issues, and manage complexities and ambiguities to adapt to changing contexts with agility (MOE, 2023, p.8). Thus, this project aims to help teachers see how similar tasks on the textbook and through simulations can achieve the same learning outcome when blended appropriately and when facilitated effectively.
An example of a thinking task in Primary 1B textbook (p.39):
|
Textbook Task: |
Prototype of a simulation: |
Textbook Task:
Prototype of a simulation:
Example of guided questions to prompt metacognitively:
What is the fastest way to find out how many ways of packing the sweets into the bags?
How to find the number of sweets in the last bag? Do you use addition only? Subtraction only? Can you use addition or subtraction? Which way do you prefer and why?
https://sg.iwant2study.org/ospsg/index.php/interactive-resources/mathematics new ones made by sithu and kangrui
In the above example, students could either draw or use physical manipulatives in attempting the task. However, with the simulation, students could use the virtual manipulatives (by clicking and dragging) and trial multiple times. For students who are not able to find a strategy to do it the fastest way with 9, on the simulation, they could vary the numbers and attempt a smaller number e.g. 4 or 6 sweets. If a student is able to observe a pattern or strategy, they can also confirm it by varying the number of sweets and number of bags. This approach allows students to explore various methods of solving the problem without being overwhelmed by too many physical manipulatives around them.
Tapping on the expertise of a primary mathematics curriculum specialist and an educational technology specialist, this project aims to collaboratively learn together and develop lesson plans that enhance students’ learning experience and develop teachers’ e-pedagogical skills.
RESEARCH METHODOLOGY AND DESIGN (Provide a brief description of your intended research design, including sampling, data collection and data analysis methods and plans)
Participants and Tasks :
Sample: A convenient sample of 3 classes of students from Primary 2 to 4 (preferably 1 class from each level). These classes will be selected from teachers who volunteer to participate in the study.
Access: Both teachers and students will be given access to the simulations in the SLS lessons.
Task Timing: Since the thinking tasks are located at the end of each topic in the textbooks, students will attempt the tasks only after completing each topic.
Task Attempts: Each class will attempt 2 tasks in total, with 1 task per topic.
Data Collection and Analysis:
Design: A mixed-method research design will be adopted for this study. Quantitative data (e.g., survey inventories and data on students’ simulation attempts) and qualitative data (e.g., feedback from teachers and students) will be collected.
Example of data on students’ simulation attempts collected building on a 2021 SSTRF from ETD
Difficulty Levels: As each simulation will have varying levels of difficulty, data will be collected on the number of sub-tasks (categorized by difficulty level) that each student attempts.
Student Survey: At the end of each task, students will complete a survey consisting of both Likert scale items and qualitative self-report segment. This survey will assess how the simulation has helped them learn the topic and suggest improvements to the simulation’s context, representation, interactions, feedback, and scaffolding.
Teacher Survey: Teachers will also complete a survey with Likert scale items and self-report segments to determine how they use the data collected from the tasks to facilitate classroom discussions, foster CAIT, and develop students’ metacognition in mathematics.
Discussion Sessions: Discussion sessions based on the thinking tasks will be video recorded to provide insights into how teachers engage students in discussions about the tasks.
Quantitative Analysis: Descriptive statistics will be compiled for the survey inventory items and the data on students’ simulation attempts.
Qualitative Analysis: The qualitative elements from the surveys, observations by researchers, and video recordings will provide a detailed description of the simulation-based lessons. These qualitative insights will complement the quantitative results, deepening our understanding of how such lessons are conducted.
UTILITY OF RESEARCH & DELIVERABLES (State how your proposed research will improve existing policy and/or practice)
As educational technology gets pervasive in the classroom, the proposed research will provide a better understanding of implementing simulation-based mathematics lessons. While these simulation-based lessons are designed to foster thinking and developing students’ metacognition in mathematics, it can also be easily repurposed in SLS for self-directed learning.
- PROJECT IMPLEMENTATION SCHEDULE (Gantt chart format)
|
Milestones |
Month |
|||||||||||
|
Jan |
Feb |
Mar |
Apr |
May |
Jun |
Jul |
Aug |
Sep |
Oct |
Nov |
Dec |
|
|
Literature scan on educational simulation |
✅︎ |
✅︎ |
||||||||||
|
Develop simulation based on thinking tasks in the textbooks |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
|
|
Trial in schools |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
||||||
|
Improvement to simulation |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
✅︎ |
||||
|
Prepare final report |
✅︎ |
✅︎ |
||||||||||
MX 13 (I) officers (or equivalent) Polytechnic Diploma / Full GCE ‘A’ Level Certificate Polytechnic Diploma Holder
To translate the task in textbook to simulations, assist in survey and interview questions and data sense making, administering tasks in schools, discussion with experts and reporting writing.
https://weelookang.blogspot.com/2025/01/2025-sstrf-use-of-digital-simulation.html
- Details
- Category: Projects
- Hits: 1456