Translations
| Code | Language | Translator | Run | |
|---|---|---|---|---|
 |
||||
Credits



This email address is being protected from spambots. You need JavaScript enabled to view it.; Francisco Esquembre; Felix J. Garcia Clemente; concept by Boon Chien Yap
Older Version
Translations
| Code | Language | Translator | Run | |
|---|---|---|---|---|
 |
||||
Credits



This email address is being protected from spambots. You need JavaScript enabled to view it.; Francisco Esquembre; Felix J. Garcia Clemente; concept by Boon Chien Yap
Briefing Document: Balancing Act Modeling Instruction
Date: October 26, 2023 Subject: Review of Sources on "Balancing Act Modeling Instruction"
1. Introduction:
This briefing document summarizes the main themes and important ideas presented in the provided excerpts related to "Balancing Act Modeling Instruction." The sources consist of a title referencing "Balancing Act Modeling Instruction" and an excerpt from the "Moment of Balancing Beam with Modelling Instruction approach by Boon Chien" page from Open Educational Resources / Open Source Physics @ Singapore. These sources detail a pedagogical approach using a balancing beam simulation to teach the concept of moment of force (torque) and the principle of moments within a Modeling Instruction framework.
2. Main Themes and Important Ideas:
- Modeling Instruction Approach: The central theme across both sources is the application of Modeling Instruction to teach physics concepts, specifically the "turning effects of forces" using a balancing beam as a model. The excerpt from Boon Chien explicitly states "Moment of Balancing Beam with Modelling Instruction approach."
- Concept of Moment of Force (Torque): A primary learning goal is to enable students to "understand the concept of moment of a force (torque)." The definition is provided as the "product of force and perpendicular distance from the line of action of the force to a fixed point (pivot)."
- Principle of Moment: The activity aims for students to "apply the establish Principle of Moment for body in static equilibrium." This principle is described as "clockwise moment = anticlockwise moment" being the "first condition for balance/equilibrium."
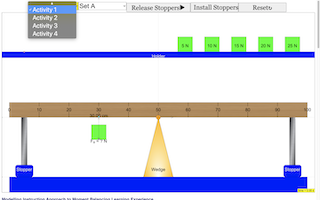
- Interactive Simulation: The core of the learning activity revolves around an interactive JavaScript simulation applet ("balancingact02_Simulation.xhtml" and an older version "balancingact01_Simulation.xhtml") embedded within a webpage. This allows students to conduct virtual experiments. The excerpt provides embed codes for these simulations.
- Structured Learning Activities: The lesson is structured into activities designed for model development. "Activity 1: Moment Lab MODEL DEVELOPMENT" involves students in "a scientifically oriented question," data collection and analysis ("collect, tabulate and analyse data"), and "evidence-based discussion through whiteboarding."
- Guided Inquiry: The "Turning Effect of a single weight" section outlines a guided inquiry process. Students are prompted to "Observe" the behavior of a ruler with an unknown weight, provide a possible "Explanation," and then follow "on-screen instructions on the VLA" (Virtual Learning Activity) to balance the ruler with different weights.
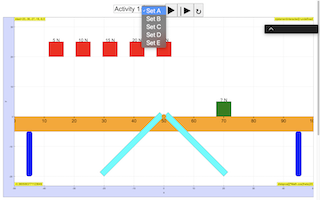
- Data Analysis and Graphical Representation: Students are required to "Record the values of the Force (F) and Distance (d) in the Google Sheet provided" and "Plot a graph of F against 1/d." This encourages mathematical reasoning and the identification of relationships between variables.
- Digital Whiteboarding and Collaborative Discussion: A significant aspect of the Modeling Instruction approach is student collaboration and communication of their findings. The sources highlight "evidence-based discussion through whiteboarding" and a "Whiteboard Meeting on the Moment Lab Model Development" where students "formulates explanations from evidence," "connects explanations to scientific knowledge," and "communicates and justifies explanations."
- Connecting Gradient to Physical Concept: The whiteboard discussion points include the "Moment of force (from the gradient of the graph of F against 1/d )" and the realization that "The value of the gradient (unit: Nm) is the same as the value of the given unknown weight x distance from the pivot." This helps students connect mathematical representations to physical meaning.
- Teacher Guidance and Resources: The "For Teachers" section provides instructions on using the Virtual Learning Activity and outlines the different activities. The inclusion of a "Sample Learning Goals and SLS Lesson" link suggests a broader curriculum integration. A video explanation by "Mr Yap" is also provided as a resource.
- Credits and Licensing: Both sources provide credits to This email address is being protected from spambots. You need JavaScript enabled to view it.; Francisco Esquembre; Felix J. Garcia Clemente; and Boon Chien Yap for the concept and compilation. The "Balancing Act Modeling Instruction" document explicitly mentions a license, and the OER Singapore page notes that "Contents are licensed Creative Commons Attribution-Share Alike 4.0 Singapore License."
3. Key Quotes:
- (Moment of a force definition): "...relate moment of force as the product of force and perpendicular distance from the line of action of the force to a fixed point (pivot)" (Moment of Balancing Beam with Modelling Instruction approach).
- (Principle of Moment): "...in order for the ruler to be balanced, clockwise moment = anticlockwise moment principle of moment (the first condition for balance/equilibrium)" (Moment of Balancing Beam with Modelling Instruction approach).
- (Model Development Activity): "Activity 1: Moment Lab MODEL DEVELOPMENT Students engage in a scientifically oriented question." (Moment of Balancing Beam with Modelling Instruction approach).
- (Whiteboard Discussion Point): "Points of discussion for Whiteboard Meeting Moment of force (from the gradient of the graph of F against 1/d )" (Moment of Balancing Beam with Modelling Instruction approach).
- (Gradient Interpretation): "The value of the gradient (unit: Nm) is the same as the value of the given unknown weight x distance from the pivot." (Moment of Balancing Beam with Modelling Instruction approach).
4. Conclusion:
The provided sources highlight a structured and interactive approach to teaching the concept of moment and the principle of moments using a balancing beam simulation within a Modeling Instruction framework. The emphasis is on student-led investigation, data analysis, collaborative discussion, and the development of conceptual understanding through evidence-based reasoning. The inclusion of virtual experiments, guided activities, and teacher resources indicates a comprehensive pedagogical design aimed at engaging students in the process of scientific model building.
Balancing Act and Moment of Force Study Guide
Quiz
- Define the term "moment of a force" (torque) in the context of rotational motion. How is it different from a linear force?
- Explain the relationship between the magnitude of a force, the perpendicular distance from the pivot, and the resulting moment. Provide an example.
- State the Principle of Moments. How is this principle applied to determine if a body is in static equilibrium?
- In the "Moment Lab" activity, students plot a graph of Force (F) against 1/distance (1/d). What does the gradient of this graph represent? What are its units?
- According to the provided text, what is the purpose of the "digital Whiteboarding" sessions in the Moment Lab activity? What skills are students expected to develop through this process?
- Describe the initial observation when the stoppers are released in the "Turning Effect of a single weight" experiment. What does this observation indicate about the forces acting on the ruler?
- What is meant by the "line of action of the force" when calculating the moment? Why is the perpendicular distance from the pivot to this line important?
- Explain the condition for rotational equilibrium in terms of clockwise and anticlockwise moments. How does this relate to the overall balance of an object?
- The text mentions that the intercept of the F against 1/d graph "may not be zero." What could be a potential reason for a non-zero intercept in this experiment?
- How does the "Modelling Instruction approach" seem to be implemented in the described activities, particularly in the Moment Lab? Provide specific examples from the text.
Quiz Answer Key
- The moment of a force (torque) is the turning effect of a force about a pivot point. Unlike a linear force that causes translational motion, a moment causes rotational motion. It depends not only on the magnitude of the force but also on its distance from the pivot.
- The moment of a force is directly proportional to both the magnitude of the force and the perpendicular distance from the line of action of the force to the pivot. For example, a larger force or a force applied further from the pivot will produce a larger moment, resulting in a greater turning effect.
- The Principle of Moments states that for a body in static equilibrium, the sum of the clockwise moments about a pivot point is equal to the sum of the anticlockwise moments about the same pivot point. This principle is used to analyze balanced systems where there is no net rotation.
- The gradient of the graph of Force (F) against 1/distance (1/d) represents the moment of the unknown weight. The units of the gradient are Newton-meters (Nm).
- Digital Whiteboarding sessions encourage students to collaboratively organize, analyze, and discuss the data they collected during the Moment Lab. Through this process, students develop skills in formulating explanations from evidence, connecting those explanations to scientific knowledge, and communicating and justifying their findings.
- When the stoppers are released, the metre ruler with the unknown weight hanging on one side turns, typically anticlockwise if that weight is initially further from the ground. This observation indicates that the gravitational force on the unknown weight is creating a moment about the pivot, causing the rotation.
- The line of action of the force is an imaginary line extending indefinitely along the direction of the force. The perpendicular distance is the shortest distance from the pivot to this line of action. This perpendicular distance is crucial because only the component of the force acting perpendicularly to the lever arm contributes to the moment.
- For rotational equilibrium, the total clockwise moment acting on an object about a pivot must be equal in magnitude to the total anticlockwise moment acting about the same pivot. This balance of moments ensures that there is no net turning effect, and the object remains in rotational equilibrium.
- A non-zero intercept on the F against 1/d graph could suggest the presence of a systematic error in the experiment, such as an offset in the distance measurements or a small initial imbalance in the ruler itself that requires a non-zero force even at theoretically infinite distance to compensate.
- The Modelling Instruction approach is evident through the emphasis on student-led investigation (Moment Lab), data collection and analysis, evidence-based discussions (digital Whiteboarding), and the development of a conceptual model (the Principle of Moments) from these experiences. The activities guide students to "discover" the concept of moment through experimentation and collaborative sense-making, rather than directly presenting the formula or principle.
Essay Format Questions
- Discuss how the "Moment Lab" activity, with its focus on data collection, graphing, and digital whiteboarding, facilitates the development of students' understanding of the concept of moment of a force and the Principle of Moments using the Modelling Instruction approach.
- Explain the significance of the Principle of Moments in analyzing systems in static equilibrium. Provide real-world examples where understanding this principle is crucial for design and safety.
- Critically evaluate the strengths and limitations of using a virtual lab simulation, as described in the texts, for teaching the concept of the moment of a force compared to a traditional hands-on laboratory experiment.
- The "Turning Effect of a single weight" experiment serves as an initial exploration. Discuss how this simple setup helps students build a qualitative understanding of torque before moving on to quantitative measurements and the formulation of the Principle of Moments in the "Moment Lab."
- Analyze the different elements of the provided materials (simulation, learning goals, teacher instructions, whiteboard discussions) and how they collectively contribute to a coherent and effective learning experience based on the principles of Modelling Instruction.
Glossary of Key Terms
Moment of a Force (Torque): The turning effect of a force about a pivot point. It is calculated as the product of the force and the perpendicular distance from the line of action of the force to the pivot.
Pivot: The fixed point around which an object can rotate. Also known as the fulcrum or axis of rotation.
Line of Action of Force: An imaginary line extending indefinitely along the direction in which the force is acting.
Perpendicular Distance: The shortest distance from the pivot point to the line of action of the force. This distance is measured along a line perpendicular to the line of action of the force.
Static Equilibrium: A state in which an object is not undergoing any translational or rotational acceleration. For rotational equilibrium, the net torque acting on the object is zero.
Principle of Moments: For a body in static rotational equilibrium, the sum of the clockwise moments about any pivot point is equal to the sum of the anticlockwise moments about the same pivot point.
Modelling Instruction: An instructional approach that emphasizes the development and application of conceptual models through student-centered activities, data analysis, and collaborative discussion.
Digital Whiteboarding: A collaborative online environment where students can share, discuss, and refine their ideas, data, and explanations, often using digital tools to represent their thinking visually.
Gradient of a Graph: The slope of a line on a graph, calculated as the ratio of the change in the vertical axis to the change in the horizontal axis. In the context of the Moment Lab, the gradient of the F vs 1/d graph represents the moment.
Turning Effect: The tendency of a force to cause rotation about a pivot. The magnitude of the turning effect is quantified by the moment of the force.
Sample Learning Goals and SLS Lesson
- understand the concept of moment of a force (torque)
- relate moment of force as the product of force and perpendicular distance from the line of action of the force to a fixed point (pivot)
- apply the establish Principle of Moment for body in static equilibrium
https://vle.learning.moe.edu.sg/my-library/lesson/view/20ceeafb-7a0c-49e6-b82d-0128b5bc18bb
For Teachers
Instruction for using the VLA for the various activities
- Please open the VLA on a new tab.
- Select Activity
- Select Set Number (if applicable)
- Select the Release/Install Stoppers Button in between loading and unloading of the weights
- Keep this tab open for the next few virtual experiments.
-
Activity 1: Moment Lab
-
MODEL DEVELOPMENT
- Students engage in a scientifically oriented question.
- Students collect, tabulate and analyse data from the investigation.
- Students involved in evidence-based discussion through whiteboarding.
Turning Effect of a single weight
Look closely at the setup below. There is an unknown weight hanging on a ruler which is pivoted at its centre. The ruler is supported by two stoppers.Observe what happen to the ruler in Figure 1 when the stoppers are released.What caused the ruler to behave this way? Write down a possible Explanation below.Answer: The metre ruler turns (anticlockwise), the left hand side side of the ruler with the unknown weight is nearer to the ground.Procedure- Follow the on-screen instructions on the VLA. Try to balance the ruler with the different weights provided.
- Record the values of the Force (F) and Distance (d) in the Google Sheet provided under your group number tab.
- Plot a graph of F against 1/d. (The graph will be generated by the Google Sheet as the points are being tabulated.)
- Discuss with your group members how to organise the data collected to support the findings from the group. (This process of collective thinking and collaboration is known as digital Whiteboarding)
-
Whiteboard Meeting on the Moment Lab
-
Model Development
- Students formulates explanations from evidence
- Students connects explanations to scientific knowledge
- Students communicates and justifies explanations
Points of discussion for Whiteboard Meeting- Moment of force (from the gradient of the graph of F against 1/d )
- identification of the correct pair of force and its corresponding perpendicular distance to the pivot, and the discussion points from the graphs
- Straight line graph
- Intercept may not be zero
- Units of axes
- Units of gradient and intercept
- At least two groups have the same values for gradient (and intercept); why?
- The rotation of the ruler due to the added weight (external force) is opposite to that given. In other words, one side the force tend to turn the ruler in clockwise direction and on the other side anticlockwise direction
- The value of the gradient (unit: Nm) is the same as the value of the given unknown weight x distance from the pivot.
- We give the name of this concept: Moment or Turning Effect of force or Torque
- In order for the ruler to be balanced, clockwise moment = anticlockwise momentprinciple of moment (the first condition for balance/equilibrium)
Research
[text]
Video
https://youtu.be/l2Udyp4ujBY Video Explanation by Mr Yap on Moment of Balancing Beam with Modelling Instruction approach
Version:
- http://weelookang.blogspot.com/2020/06/moment-of-balancing-beam-with-modelling.html
- https://vle.learning.moe.edu.sg/my-library/lesson/view/20ceeafb-7a0c-49e6-b82d-0128b5bc18bb
Other Resources
[text]
Frequently Asked Questions: Balancing Act and Moment of Force
1. What is the primary concept explored in the "Balancing Act Modeling Instruction" and "Moment of Balancing Beam" resources?
The primary concept explored is the moment of a force, also known as torque. These resources utilize a balancing beam scenario to help learners understand how forces applied at a distance from a pivot point can create a turning effect. The goal is to develop a conceptual understanding of what causes rotation and how to achieve rotational equilibrium.
2. What is "Modeling Instruction" in the context of these resources?
"Modeling Instruction" is an approach where students actively engage in scientific inquiry to develop, test, and refine conceptual models. In the "Moment Lab" activity described, students don't just passively receive information; instead, they conduct virtual experiments, collect and analyze data, formulate explanations based on evidence, and engage in discussions (digital whiteboarding) to collaboratively construct their understanding of the moment of force.
3. What is the definition of the moment of a force (torque) according to these resources?
The moment of a force (torque) is defined as the product of the force and the perpendicular distance from the line of action of the force to a fixed point (the pivot). This means both the magnitude of the force and how far it is applied from the pivot, and at what angle, determine its turning effect.
4. What is the "Principle of Moment" and how does it relate to a balanced beam?
The "Principle of Moment" states that for a body to be in static equilibrium (balanced and not rotating), the total clockwise moment about a pivot must be equal to the total anticlockwise moment about the same pivot. This principle is central to understanding how to balance the beam by adjusting the forces (weights) and their distances from the pivot.
5. How do the provided virtual simulations aid in learning about the moment of force?
The virtual simulations allow students to experiment with different weights and their positions on a balancing beam in a controlled environment. They can observe the turning effects of forces, collect quantitative data (force and distance), and analyze the relationship between these variables. This hands-on virtual experience helps them visualize abstract concepts and test their hypotheses, leading to a deeper understanding of the moment of force and the principle of moment.
6. What is the significance of the graph of Force (F) against 1/distance (1/d) in the "Moment Lab" activity?
Plotting a graph of Force (F) against 1/distance (1/d) is a technique used to linearize the relationship between these variables as it relates to the moment of force (Torque = F * d). If the moment (Torque) is constant, then F is inversely proportional to d, meaning F is directly proportional to 1/d. The gradient of this graph represents the moment of the unknown weight, allowing students to determine its turning effect quantitatively.
7. What is the role of "digital Whiteboarding" in the learning process described in these resources?
Digital whiteboarding is a collaborative activity where students discuss their observations, data, and interpretations in a shared virtual space. This process encourages them to articulate their understanding, share evidence, debate findings, and collectively construct explanations for the phenomena they observe in the simulations. It fosters communication, critical thinking, and the development of a shared mental model of the concept.
8. Besides balancing beams, where else might the concept of the moment of force (torque) be applicable in physics and real-world scenarios?
The concept of the moment of force (torque) is fundamental in many areas of physics and has numerous real-world applications. These include:
- Rotational Motion: Understanding how objects rotate, accelerate, and come to rest due to applied torques.
- Simple Machines: Analyzing the mechanical advantage of levers, wrenches, and other tools that utilize torque.
- Engineering: Designing structures and mechanisms that can withstand or produce rotational forces, such as engines, motors, and bridges.
- Everyday Life: Understanding how doors open and close, how we steer a car, or how a see-saw works are all examples of torque in action.
- Advanced Physics: Torque is crucial in understanding angular momentum, gyroscopic motion, and the behavior of rotating systems at various scales.