About
The Art of Symmetry - Structured Beauty in Nature
To many of us, whenever someone mentions the word "symmetry", the first thing that comes to mind is some shape that looks the same when reflected in a mirror. To some, yet another thing that comes to mind would be some form of balance and harmony, like the Yin and Yang of Taoist culture.

Symmetry is a property of objects best described in the mathematical lingo as "the property of staying invariant under some action". Those are big words, so let us try to break down what they intuitively mean. The word "invariant" is just a fancy, more technical term for "unchanged", and in mathematics, the word "action" can be thought of as something like a reflection, a rotation, a translation, et cetera.
In other words, symmetry simply refers to the property of some object being unchanged by some reflection, rotation or translation (there is more subtlety and is much more general than that, but we will not go there as it is beyond the scope of this text). Take a moment to ponder this interpretation of what symmetry means, and try to imagine how this does indeed capture the intuition that we have regarding symmetrical objects.
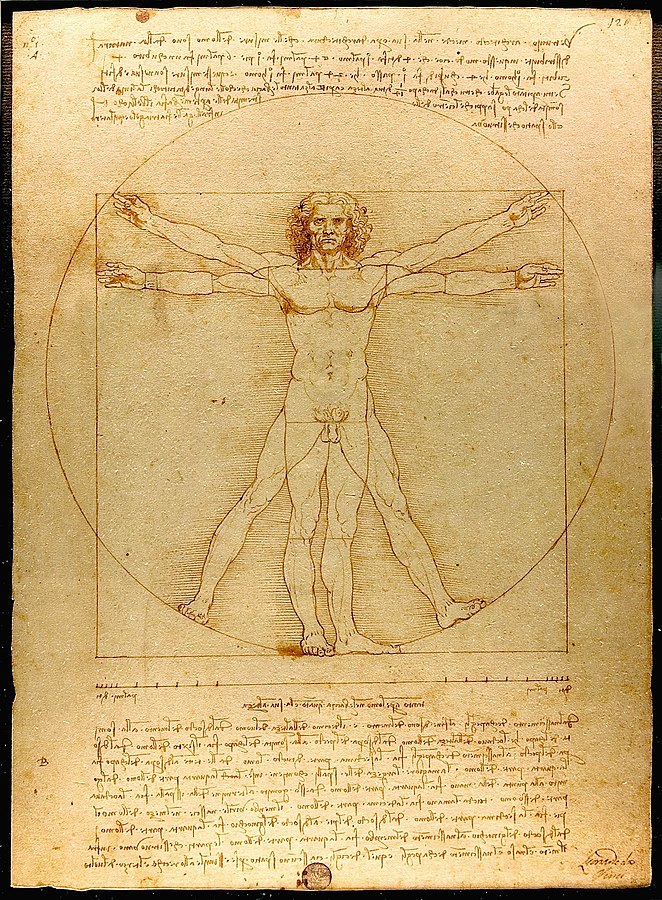
As the title of this chapter implies, symmetry is seen as a form of art, and is found everywhere in nature. Look around you, and you can see symmetries in flowers, the moon, and in the appearances of many animals. In fact, humans are mostly symmetric too! The famous Italian polymath Leonardo da Vinci was well-known for drawing the Vitruvian Man, what he thought was the ideal proportions of the human body. One thing that stands out in the Vitruvian Man is the high level of symmetry, which says a lot about how symmetry is viewed as an ideal form of beauty in society. Think about it, we humans tend to see other people as more beautiful and attractive if their faces and bodies are symmetric.
Symmetry shows up all the time in architecture as well, with the Taj Mahal and the Islamic design of mosques being prime examples of buildings with very symmetrical designs. It would be no surprise that we humans use the same measure of symmetry to judge the beauty of both buildings and other living things. After all, there is some elegance to symmetry, no?

Well, at this point, we are getting ahead of ourselves. All this talk about symmetry, and we still have not actually discussed what exactly it is we are talking about. Without further ado, let us then proceed to the first chapter.
Chapter 1: Mirror Mirror On The Wall, Who Is The Most Symmetrical Of Them All?
A vain queen strolls down the steps of her castle, down into the deep, dark dungeon. Entering her own private workshop, she dusted off a tall object draped in a shabby cloth, and with a well-practiced flourish, pulled the cloth away to reveal a beautiful mirror.
"Mirror Mirror on the wall."
The screen of the mirror suddenly sprung to life, the various colors of smoke coming together in harmony to form a rainbow-colored mask. Three holes cut themselves into the mask of smoke, forming what appeared to be a very simplified face.
"How may I help you today, my queen?"
The queen, unshaken by this colorful display in front of her, coldly replied with a line that she must have said many times before.
"Who is the most symmetrical of them all?"
"Of course, it is you, my queen."
Now, the magic mirror did not believe one bit of what it said, of course. Being based off an object that is designed to look the same in mirror reflections, it is clearly much more symmetrical than the human-shaped queen. However, she is the queen of the land, and the mirror is bound to her service, and so it must satisfy her.
Satisfied with the day's answer, the queen left the dungeons, leaving the mirror to its own thoughts. It was troubled by the recent appearance of several, more symmetric beings in the kingdom, and seeing that the queen did not enjoy the presence of beings more symmetrical than herself, the mirror did not know what to tell the queen.
The next day, the queen descended into the dungeon again, and asked the same question as she had always asked for the past many years.
"Mirror Mirror on the wall, who is the most symmetrical of them all?"
This time, the mirror hesitated. This behavior was most strange, for the mirror usually replies in an instant.
"Of course you are a very symmetrical being, my queen. But..." the mirror seemed troubled, "there have appeared beings who are more symmetrical than you are, my queen."
Upon hearing this, the air around the vain queen shifted. In a fit of silent rage, she swiftly left the dungeon, back into her throne room. She summoned the foreign, supposedly more symmetrical beings to her castle the next day.
"Hear me, foreign ones. I am the queen of this land, most symmetrical of all."
The entourage of strange beings, whose shapes were not humanoid in the least, simply remained where they were, completely unfazed by the regal display before them.
"I will not allow beings who are more symmetrical than I to stay in my kingdom. But I am a fair ruler, and I shall give you all one chance to prove the extent of your symmetry."
The queen then motioned to a member of her court to step forward.
"Now come before the judge, foreign beings, and prove to everyone else your lack of symmetry!"
In the following simulation, we shall explore the concept of reflection symmetry.
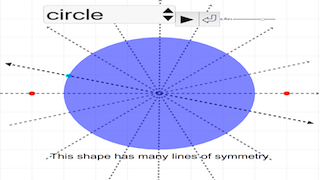
Objects which have reflection symmetry have this special thing called a line of symmetry. Simply put, a line of symmetry is a line with the property that if you cut or fold the object along the line, the resulting two halves are exactly mirror images of each other. Another way to describe a line of symmetry, keeping with our theme of mirrors in this chapter, is that a mirror which reflects an object across its line of symmetry will leave the object looking exactly the same, as if it has never been reflected.
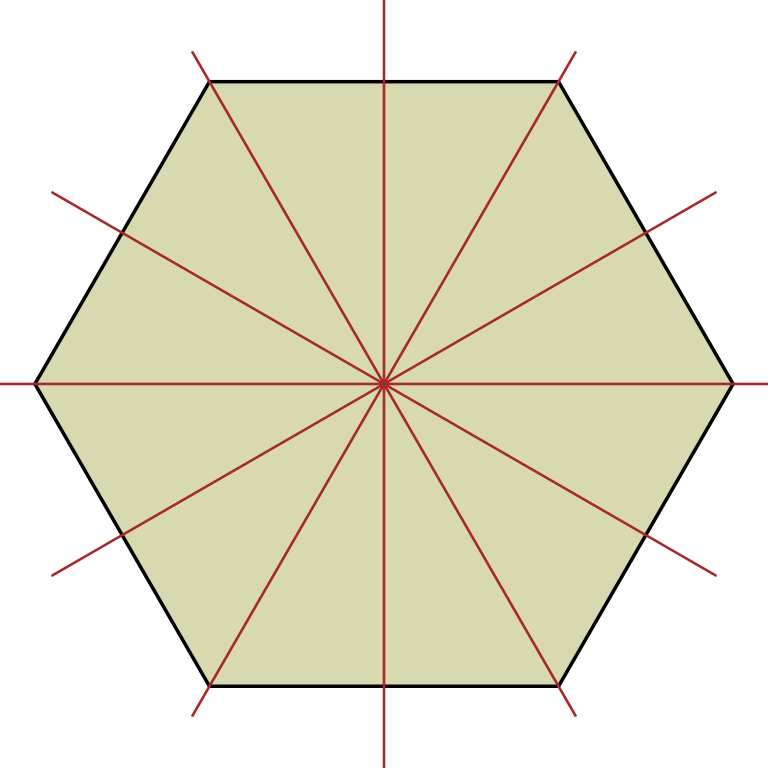
Of course, there is nothing stopping an object from having multiple lines of symmetry. In fact, as we will see later, we can find objects with as many lines of symmetry as we want!
The foreign beings, whose bodies take the form of various shapes, line up one by one in front of you to check how symmetric they are. You, as the judge, will try to determine if they have reflection symmetry or not, and if so, how many lines of symmetry they have. Fret not, the queen has patience, and will wait for as long as you need to conduct the check. Have fun!
Translations
| Code | Language | Translator | Run | |
|---|---|---|---|---|
 |
||||
Credits


 This email address is being protected from spambots. You need JavaScript enabled to view it.; Francisco Esquembre; Felix J. Garcia Clemente
This email address is being protected from spambots. You need JavaScript enabled to view it.; Francisco Esquembre; Felix J. Garcia Clemente
Briefing Document: "Mirror Mirror On The Wall, Who Is The Most Symmetrical Of Them All?"
1. Introduction
This document provides a summary and analysis of the interactive resource titled "Chapter 1: Mirror Mirror On The Wall, Who Is The Most Symmetrical Of Them All? Symmetry Shape JavaScript HTML5 Applet Simulation Model," hosted on the Open Educational Resources / Open Source Physics @ Singapore website. This resource uses a narrative and an interactive simulation to explore the concept of symmetry, specifically reflection symmetry, in an engaging way.
2. Main Themes
- Definition of Symmetry: The core theme is the exploration of symmetry as a mathematical property of objects, defined as being "invariant under some action." The document clarifies that "invariant" means "unchanged," and "action" refers to operations like reflection, rotation, or translation. It states, "Symmetry is a property of objects best described in the mathematical lingo as 'the property of staying invariant under some action'."
- Reflection Symmetry (Line Symmetry): The chapter specifically focuses on reflection symmetry, introducing the concept of a "line of symmetry". It explains, "a line of symmetry is a line with the property that if you cut or fold the object along the line, the resulting two halves are exactly mirror images of each other." The resource highlights that objects can have multiple lines of symmetry.
- Symmetry as Art and Beauty: The document emphasizes the aesthetic appeal of symmetry, linking it to ideas of balance and harmony. It states, "symmetry is seen as a form of art, and is found everywhere in nature." The document mentions examples of symmetry in nature, including "flowers, the moon, and in the appearances of many animals," as well as in human forms, like Da Vinci's Vitruvian Man and in architecture like the Taj Mahal, with the implication that these are seen as beautiful because of their symmetry.
- Symmetry in Human Perception: The document connects symmetry to how humans perceive beauty and attractiveness, noting, "we humans tend to see other people as more beautiful and attractive if their faces and bodies are symmetric." It implies a link between symmetry and the perception of attractiveness and perfection.
- Exploration Through Simulation: The document introduces an interactive element where the user acts as a judge, examining different shapes to determine if they possess reflection symmetry and how many lines of symmetry each has.
3. Key Ideas and Facts
- Mathematical Definition of Symmetry: Symmetry is not merely about looking the same but about remaining unchanged under specific transformations. The text stresses the formal definition of symmetry as invariance under actions.
- Multiple Types of Symmetry: Although the focus is on reflection symmetry, the text mentions rotational and translational symmetries, acknowledging the concept of symmetry is much broader than just mirror reflections.
- Line of Symmetry as a Mirror: The explanation of a line of symmetry as a mirror that leaves the object unchanged upon reflection is a key concept for understanding the interactive simulation.
- The Story of the Vain Queen: The narrative of a vain queen who seeks to be the most symmetrical sets the stage for exploring symmetry and creates an engaging context for the interactive simulation. This story uses the idea of a queen to introduce the idea of a hierarchy of symmetry.
- Interactive Learning: The simulation component encourages hands-on learning and promotes understanding of reflection symmetry through user engagement. The user acts as judge, evaluating the symmetry of presented shapes.
4. Illustrative Quotes
- On the meaning of symmetry: "Symmetry is a property of objects best described in the mathematical lingo as 'the property of staying invariant under some action'."
- On reflection symmetry: "Simply put, a line of symmetry is a line with the property that if you cut or fold the object along the line, the resulting two halves are exactly mirror images of each other."
- On the connection to beauty: "Think about it, we humans tend to see other people as more beautiful and attractive if their faces and bodies are symmetric."
5. Educational Purpose
The resource appears to be designed for educational purposes, particularly to teach students about the concept of symmetry, particularly reflection symmetry. The use of a narrative, clear definitions, and interactive simulation makes it a user-friendly way to explore this topic, which could potentially benefit from more creative educational materials.
6. Interactive Element
The simulation is not detailed in the provided text beyond the description of its purpose. However, it's clear that the interactive component is the main way for users to actively explore reflection symmetry.
7. Conclusion
The "Mirror Mirror on the Wall" resource provides a good introduction to the concept of symmetry through a combination of narrative, explanation, and interactive simulation. By linking the concept to both mathematics and the perception of beauty, the resource provides a comprehensive and relatable learning experience. It effectively introduces and allows the user to learn about reflection symmetry through its simulation. The document also suggests that, through its other linked resources, this is part of a broader series of educational simulations and materials.
Symmetry Study Guide
Quiz
Instructions: Answer each question in 2-3 sentences.
- What is the definition of symmetry according to the text?
- What does the term "invariant" mean in the context of symmetry?
- Besides reflection, what other types of actions are mentioned in relation to symmetry?
- How does the text relate the concept of symmetry to beauty?
- What is a line of symmetry?
- In the story, why does the mirror initially tell the queen that she is the most symmetrical?
- What causes the mirror to change its answer to the queen's question about symmetry?
- In the simulation, what role does the user play?
- According to the text, is it possible for an object to have multiple lines of symmetry?
- What is the basic premise of the interactive simulation described in the text?
Quiz Answer Key
- Symmetry is defined as the property of an object remaining unchanged (invariant) under certain actions such as reflection, rotation, or translation. It is a mathematical concept describing how an object can maintain its appearance after undergoing a specific transformation.
- In the context of symmetry, "invariant" means unchanged. It refers to a property of an object that remains the same even when the object is acted upon by transformations such as reflection, rotation, or translation.
- Besides reflection, the text mentions rotation and translation as actions that can demonstrate symmetry. These actions are ways in which an object can be transformed while still retaining its original properties and appearance.
- The text suggests that symmetry is often associated with beauty and attractiveness. It implies that humans tend to find symmetrical faces and bodies more appealing, thus equating symmetry to an ideal form of beauty.
- A line of symmetry is a line that, when an object is cut or folded along it, creates two halves that are mirror images of each other. Alternatively, if a mirror is placed along this line and reflects the object, it appears identical to its original state.
- The mirror initially tells the queen that she is the most symmetrical because it is bound to her service and seeks to satisfy her, despite not believing it. As the queen of the land, she expects to be told she is the most beautiful and, by extension, symmetrical.
- The mirror changes its answer because it has noticed the presence of other beings in the kingdom that are, in fact, more symmetrical than the queen. It can no longer deny the existence of these more symmetrical beings, causing its initial lie to be revealed.
- In the simulation, the user plays the role of a judge tasked with determining whether objects have reflection symmetry and how many lines of symmetry they possess. The user evaluates shapes to decide how symmetrical they are.
- Yes, the text explicitly states that objects can have multiple lines of symmetry. The number of lines of symmetry can vary, and some objects may even have an infinite number of lines of symmetry.
- The interactive simulation explores the concept of reflection symmetry, allowing users to determine if presented shapes have symmetry and to find the number of lines of symmetry for those objects. The user makes assessments about the various shapes presented.
Essay Questions
- Discuss the concept of symmetry as presented in the text, drawing connections between its mathematical definition and its aesthetic appeal. How does the text use examples from nature and human art to illustrate these connections?
- Analyze the narrative used in the text about the queen and the magic mirror. How does this story serve to introduce and explore the concept of symmetry, and what does it reveal about human perceptions of beauty and symmetry?
- The text focuses primarily on reflection symmetry, but it also briefly mentions rotational and translational symmetries. Elaborate on these different forms of symmetry using examples not explicitly mentioned in the text, and explain how each is a type of "invariance under action."
- Consider the role of interactive simulations in learning about complex concepts, such as symmetry. How might this applet enhance a learner’s understanding of symmetry, and what are the potential benefits of this type of learning tool?
- The text connects symmetry to art and architecture. Analyze this relationship by using examples of art and architecture beyond those specifically mentioned in the text. How does the incorporation of symmetry impact the aesthetic and functional characteristics of these objects?
Glossary
- Symmetry: The property of an object that remains unchanged (invariant) under certain actions like reflection, rotation, or translation. It is a mathematical property that describes how an object can maintain its appearance after undergoing a transformation.
- Invariant: A term used in mathematics to describe something that remains unchanged or constant, even when certain actions or transformations are applied. In the context of symmetry, it refers to a property that doesn't change.
- Reflection Symmetry: A type of symmetry where an object looks the same when reflected across a line, known as a line of symmetry. It is also sometimes called mirror symmetry.
- Rotation Symmetry: A type of symmetry in which an object can be rotated about a central point and remain unchanged.
- Translation Symmetry: A type of symmetry where an object can be moved or shifted in a straight line and still retain the same properties and look unchanged.
- Line of Symmetry: A line that divides an object into two mirror image halves. If an object has a line of symmetry, folding or reflecting it along this line would result in identical halves.
- Vitruvian Man: A drawing by Leonardo da Vinci that depicts a man in two superimposed positions, with his arms and legs spread apart, to illustrate ideal human proportions and symmetry.
- Taijitu: A symbol from Taoism representing the Yin and Yang, often shown as a circle divided into two swirling halves, embodying rotational symmetry.
- Tessellation: A pattern made up of repeating shapes that fit together without any gaps or overlaps; these patterns often demonstrate translational symmetry.
Sample Learning Goals
[text]
For Teachers
[text]
Research
[text]
Video
https://www.youtube.com/watch?v=I36-bO_NzEk
Version:
- http://weelookang.blogspot.sg/2017/11/symmetry-shape-javascript-html5-applet.html
- https://weelookang.blogspot.com/2021/11/symmetry-shape-javascript-html5-applet.html
Other Resources
Frequently Asked Questions About Symmetry
- What is symmetry, and how is it defined mathematically?
- Symmetry, in a general sense, is the property of an object remaining unchanged under certain actions. Mathematically, it's described as the property of staying invariant under an action. Invariant means 'unchanged', and actions can include reflections, rotations, or translations. Essentially, if you perform one of these actions on a symmetrical object, it looks the same as it did before.
- What are the common types of symmetry?
- The common types of symmetry include: * Reflection Symmetry (Line Symmetry): An object has reflection symmetry if a line can divide it into two mirror-image halves. This line is called a line of symmetry. * Rotational Symmetry: An object has rotational symmetry if it looks the same after being rotated by a certain angle around a central point. * Translational Symmetry: An object has translational symmetry if it looks the same after being moved (translated) a certain distance.
- Why is symmetry considered a form of art and found so prevalently in nature?
- Symmetry is considered a form of art because it often embodies balance, harmony, and structured beauty. In nature, symmetry is abundant, appearing in flowers, the moon, and the appearances of many animals including humans. The inherent balance and visual appeal of symmetry make it an ideal form of beauty in many cultures and is often used in art and architecture.
- How does the "Vitruvian Man" relate to the concept of symmetry?
- Leonardo da Vinci's "Vitruvian Man" is a prime example of symmetry in the human form. It portrays what Da Vinci considered the ideal proportions of the human body, which notably features a high degree of symmetry. This highlights the idea that symmetry is often perceived as an ideal of beauty. We generally find people with symmetrical features more attractive.
- How does the concept of a 'line of symmetry' work in reflection symmetry?
- A line of symmetry is a line that divides an object into two mirror-image halves. If you imagine folding the object along the line of symmetry, the two resulting halves would match exactly. Another way to look at it is if you reflect the object using the line of symmetry as a mirror, the object would appear unchanged. An object may also have multiple lines of symmetry.
- Why does the mirror in the story have trouble answering the queen's question about who is most symmetrical?
- The mirror in the story initially flatters the queen, even though it knows it's more symmetrical due to its reflective properties. It hesitates because it recognizes the emergence of more symmetrical non-human shapes in the kingdom. This conflict causes the mirror to have trouble telling the truth, as it knows the queen will not be happy knowing that there are more symmetrical beings than her.
- In the context of the simulation, what is the user asked to do?
- In the simulation, the user acts as a judge, evaluating various shapes presented to them to determine whether each shape has reflection symmetry. They also count the number of lines of symmetry for shapes that have reflection symmetry. This task allows the user to explore the concept of reflection symmetry in a hands-on way.
- Besides reflection symmetry, what other mathematical and scientific concepts are connected to symmetry?
- While the source mainly focuses on reflection symmetry, it touches on rotation and translation symmetry. More broadly, symmetry relates to concepts like invariance and transformations in mathematics. In science, symmetry can appear in different forms, like particle physics and crystallography. The principle that underlying laws of physics should remain invariant under various transformations are a crucial part of advanced physics. The concepts extend to music, architecture, and design, where different symmetries are used to create visual and auditory effects.
- Details
- Written by Fremont
- Parent Category: geometry
- Category: 3. Line Symmetry
- Hits: 10718