after hui jun inputs, simpler UI
Translations
| Code | Language | Translator | Run | |
|---|---|---|---|---|
 |
||||
Credits

Coco; lookang
Translations
| Code | Language | Translator | Run | |
|---|---|---|---|---|
 |
||||
Credits

Coco; lookang
Translations
| Code | Language | Translator | Run | |
|---|---|---|---|---|
 |
||||
Credits

Coco; lookang
Sample Learning Goals
Virtual Lab: Understanding Upthrust Using Submarine Simulation
Objective
To investigate and understand the concept of upthrust (buoyant force) acting on a submerged object using a submarine simulation.
Materials
- Computer with internet access
- Submarine simulation link: Submarine Simulation
- Lab worksheet (provided below)
Theory
Upthrust or buoyant force is the upward force exerted by a fluid that opposes the weight of an object submerged in the fluid. According to Archimedes' principle, the buoyant force is equal to the weight of the displaced fluid.
Steps
-
Initial Setup:
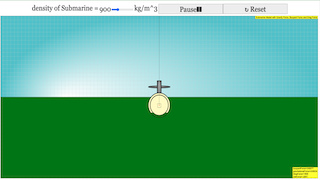
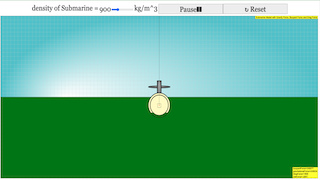
- Open the submarine simulation using the provided link Submarine Simulation.
- Familiarize yourself with the simulation interface. Note the controls for adjusting the density of the submarine and the draggable square to give the submarine velocity.
-
Observation at Rest:
- Set the density of the submarine to 1000 kg/m³, which matches the density of water.
- Observe the submarine at rest. Note its position and forces acting on it (buoyant force, gravitational force, drag force, net force).
-
Effect of Density:
- Gradually increase the density of the submarine using the slider.
- Observe and record the changes in the submarine's behavior and the forces acting on it.
- Repeat the process by decreasing the density of the submarine. Note your observations.
-
Applying Velocity:
- Drag the square to give the submarine an initial velocity upwards.
- Observe how the forces change as the submarine moves.
- Repeat the process by giving the submarine an initial downward velocity.
- Record your observations in both cases.
-
Buoyant Force \[ F_b = \rho_f \cdot V \cdot g \] Calculation:
- Pause the simulation when the submarine is fully submerged.
- Note the value of the buoyant force displayed.
- Using Archimedes' principle, calculate the buoyant force theoretically and compare it with the simulation value.
-
Equilibrium Position at velocity = 0:
- Adjust the density of the submarine until it reaches an equilibrium position where it neither sinks nor floats.
- Note the equilibrium density and corresponding forces.
- Discuss why the submarine is in equilibrium at this density.
-
Exploring Different Scenarios:
- Change the submarine’s density to values significantly lower and higher than the water density.
- Observe and record the behavior of the submarine in each case.
- Analyze the relationship between density and upthrust in these scenarios.
Analysis Questions
- Explain the concept of upthrust \[ F_b = \rho_f \cdot V \cdot g \] and its significance in the simulation.
- How does changing the density of the submarine affect its buoyancy?
- Describe the conditions under which the submarine floats, sinks, and remains suspended in the water.
- Calculate the theoretical buoyant force and compare it with the simulation data. Explain any discrepancies.
- Discuss how the net force acting on the submarine changes with its movement and density changes.
Suggested Answers to Analysis Questions
1. Explain the concept of upthrust \( F_b = \rho_f \cdot V \cdot g \) and its significance in the simulation.
**Answer:**
Upthrust, or buoyant force \( F_b \), is the upward force exerted by a fluid on a submerged object. It is given by the equation \( F_b = \rho_f \cdot V \cdot g \), where \( \rho_f \) is the fluid density, \( V \) is the volume of the displaced fluid, and \( g \) is the acceleration due to gravity. In the simulation, upthrust plays a crucial role in determining whether the submarine floats, sinks, or remains suspended. The upthrust counteracts the weight of the submarine, and its magnitude depends on the volume of the submarine submerged and the density of the surrounding fluid.
2. How does changing the density of the submarine affect its buoyancy?
**Answer:**
Changing the density of the submarine affects its buoyancy by altering the balance between the weight of the submarine and the upthrust. If the density of the submarine is less than the fluid density, the upthrust exceeds the weight, causing the submarine to float. If the density of the submarine is greater than the fluid density, the weight exceeds the upthrust, causing the submarine to sink. When the density of the submarine matches the fluid density, the upthrust equals the weight, and the submarine remains suspended in equilibrium.
3. Describe the conditions under which the submarine floats, sinks, and remains suspended in the water.
**Answer:**
- **Floats:** The submarine floats when its density is less than the density of the water. In this case, the upthrust is greater than the weight of the submarine, pushing it upwards.
- **Sinks:** The submarine sinks when its density is greater than the density of the water. Here, the weight of the submarine exceeds the upthrust, causing it to move downwards.
- **Remains Suspended:** The submarine remains suspended (neutrally buoyant) when its density is equal to the density of the water. The upthrust equals the weight, resulting in a net force of zero, and the submarine stays at its current depth.
4. Calculate the theoretical buoyant force and compare it with the simulation data. Explain any discrepancies.
**Answer:**
Theoretical Buoyant Force \( F_b \) can be calculated using the formula:
\[ F_b = \rho_f \cdot V \cdot g \]
Assuming:
- \(\rho_f = 1000 \, \text{kg/m}^3\) (density of water)
- \(V\) is the volume of the submarine (this value should be given or measured in the simulation)
- \(g = 9.81 \, \text{m/s}^2\) (acceleration due to gravity)
For example, if the volume of the submarine is \(1 \, \text{m}^3\), the theoretical buoyant force would be:
\[ F_b = 1000 \, \text{kg/m}^3 \cdot 1 \, \text{m}^3 \cdot 9.81 \, \text{m/s}^2 = 9810 \, \text{N} \]
Compare this value with the buoyant force displayed in the simulation. Discrepancies may arise due to factors like numerical approximations in the simulation, unaccounted forces (such as drag), or variations in the assumed values of volume and density.
5. Discuss how the net force acting on the submarine changes with its movement and density changes.
**Answer:**
The net force on the submarine is the vector sum of all forces acting on it, including gravitational force, buoyant force, and drag force. When the density of the submarine changes:
- **Increased Density:** The gravitational force increases, potentially leading to a larger net downward force if the density exceeds that of the water, causing the submarine to sink.
- **Decreased Density:** The gravitational force decreases, resulting in a larger net upward force if the density is less than that of the water, causing the submarine to float.
- **Movement:** As the submarine moves, drag force opposes its motion. The net force is the sum of gravitational, buoyant, and drag forces. If the submarine moves upwards, drag acts downwards, and vice versa. The net force determines the submarine’s acceleration and direction of motion. Changes in density affect the balance of forces, influencing the submarine's movement until equilibrium is reached.
These suggested answers will help students analyze and understand the principles of upthrust and buoyancy in the context of the submarine simulation.
Conclusion
Summarize your findings on the relationship between the submarine's density, buoyant force, and the conditions for equilibrium. Reflect on the importance of upthrust in real-life applications such as submarine design and marine engineering.
Notes for Teachers
- Ensure students have access to the necessary technology and provide assistance with the simulation link if needed.
- Encourage students to discuss their observations and analysis with peers to enhance understanding.
- Use the lab worksheet to guide students through the steps and ensure they record their findings systematically.
This virtual lab guide will help A-level students explore the principles of upthrust and gain practical understanding through interactive simulation.
Submarines and Upthrust
Learning Outcomes:
-
H2: 2(b)
Curriculum Emphasis:
-
Science, Technology, Society and the Environment
For Teachers
Research
Video
[text]
Version:
Other Resources
[text]
end faq
{accordionfaq faqid=accordion4 faqclass="lightnessfaq defaulticon headerbackground headerborder contentbackground contentborder round5"}
- Details
- Written by Coco Lee
- Category: Uncategorised
- Hits: 84415