About

Metalic conductor

How can a lot of loose electrons cause a metal to be a good conductor?

This is because each electron is an electric charge that is free to move. Moving electric charges can make up a current. But this is only true if they move the same way.

Due to thermal kinetic energy of the electrons and ions in the metal, these conduction electrons move in a random, disordered way, with a velocity close to 10⁶ m/s. In total, however, they have zero net velocity because their motion is not ordered.

However, if an external field is applied, then the net motion of the electrons will follow the field.

 

Translations

Code	Language		Translator	Run

Credits

Francisco Esquembre , Universidad de Murcia; Maria Jose Cano; lookang http://weelookang.blogspot.sg/

 

Briefing Document:🚄Drift Velocity in Metallic Conductors

Source: Excerpts from "Metallic Conductor and Drift Velocity JavaScript HTML5 Applet Simulation Model" on Open Educational Resources / Open Source Physics @ Singapore

Main Themes:

• Drift Velocity: The average velocity of charge carriers (typically electrons) in a conductor due to an applied electric field.
• Metallic Conduction: How the random motion of free electrons in metals becomes a net flow of charge when an electric field is applied.
• Factors Affecting Drift Velocity: Temperature, electric field strength, and the nature of the conductor.

Key Ideas and Facts:

1. Random Electron Motion: In the absence of an electric field, electrons in a conductor exhibit rapid, random motion due to thermal energy. Their net velocity is zero.

• "Due to thermal kinetic energy of the electrons and ions in the metal, these conduction electrons move in a random, disordered way, with a velocity close to 106 m/s."

1. Electric Field Imposes Drift: When an electric field is applied, a net drift velocity is superimposed on the random motion, causing a flow of charge (current) in the opposite direction to the electric field.

• "Applying a potential difference, which sets up a field, is much like creating a pressure difference in a gas. In both cases there will be a net flow of charges or gas particles respectively."

1. Drift Velocity is Slow: Drift velocity is much slower than the random thermal velocity of electrons.

• This is not explicitly stated in the excerpt but is a fundamental concept in understanding drift velocity.

1. Factors Affecting Drift Velocity:

• Temperature: Higher temperatures increase the random motion of electrons, making it harder for them to achieve a consistent drift velocity.
• "increase the temperature, this model can be used to visualize the effects of increased temperature on the vibrations of atoms fixed in their relative positions like a solid."
• Electric Field Strength: A stronger electric field results in a higher drift velocity.
• Conductor Material: The type of metal and its atomic structure influence electron mobility and thus drift velocity.

Educational Implications:

• The provided simulation model is a valuable tool for visualizing and understanding drift velocity.
• It allows students to manipulate variables like temperature and electric field strength to observe their effects on electron behavior.
• The model facilitates the connection between microscopic electron motion and macroscopic current flow.

Further Exploration:

• Investigate the relationship between drift velocity, current, and electron density.
• Explore the concept of electron mobility and how it varies across different materials.
• Research the limitations of the classical model of drift velocity and the need for quantum mechanical explanations in some cases.

Drift Velocity in Metallic Conductors

Short Answer Quiz

Instructions: Answer the following questions in 2-3 sentences each.

1. Explain how the random motion of electrons in a conductor changes when an electric field is applied.
2. Define drift velocity in the context of metallic conductors.
3. Describe the relationship between the direction of the electric field, the direction of the drift velocity, and the direction of conventional current flow.
4. How does the Easy JavaScript Simulations (EJSS) model demonstrate the concept of electron repulsion?
5. What is the purpose of the "showTrace" checkbox in the EJSS model?
6. According to the EJSS model, how does increasing the temperature affect the vibration of atoms in the conductor?
7. Explain how the EJSS model can be used to investigate the relationship between drift velocity and the time it takes for a light bulb to illuminate in a circuit.
8. Describe how the potential difference created by a battery affects the flow of charge carriers in a wire.
9. What is the Fermi velocity, and how does it relate to drift velocity?
10. Why are metals considered good conductors of electricity?

Answer Key

1. When an electric field is applied, the random motion of electrons is superimposed with a net drift velocity in the direction opposite to the electric field. Electrons still move randomly, but they have a slow, overall movement in one direction.
2. Drift velocity is the average velocity of charge carriers, typically electrons, in a conductor due to an applied electric field. It represents the net movement of electrons superimposed on their random thermal motion.
3. The electric field points from positive to negative potential. Drift velocity is in the opposite direction to the electric field, as electrons are negatively charged. Conventional current flow is in the same direction as the electric field, by historical convention.
4. The EJSS model shows that when electrons, which carry like charges, approach each other, they tend to repel, demonstrating the concept of electrostatic repulsion.
5. The "showTrace" checkbox in the EJSS model displays the path of a single electron, making it easier to visualize and deduce the average velocity of electrons, hence the drift velocity.
6. The EJSS model shows that as temperature increases, the vibrations of the atoms in the conductor become more energetic and have larger amplitudes, reflecting increased kinetic energy.
7. By reversing the electric field while the simulation is running and observing the change in drift velocity, students can relate this to the non-instantaneous lighting up of a light bulb. This suggests that the time it takes for the light bulb to turn on is related to the time it takes for the electrons to establish a net drift velocity.
8. A battery creates a potential difference between the ends of a wire, establishing an electric field within the wire. This field exerts a force on the charge carriers, causing a net flow of electrons from the negative terminal to the positive terminal of the battery.
9. Fermi velocity is the average velocity of electrons in a conductor at room temperature due to their thermal energy. It is much higher than drift velocity, which is the additional velocity imparted by an electric field.
10. Metals are good conductors because they have a large number of free electrons that are not bound to individual atoms. These free electrons can readily move under the influence of an electric field, contributing to electrical conductivity.

Essay Questions

1. Explain the relationship between drift velocity, current, and the number of charge carriers in a conductor. Use equations to support your explanation.
2. Discuss the factors affecting drift velocity in a metallic conductor, including temperature, electric field strength, and material properties.
3. Compare and contrast the motion of charge carriers in a conductor with and without an applied electric field. How does this difference in motion lead to the flow of electric current?
4. Analyze the limitations of the EJSS drift velocity model in representing real-world metallic conductors. What simplifications are made in the model?
5. Design an experiment using the EJSS model to investigate the relationship between drift velocity and the resistance of a conductor. Outline your procedure, expected results, and potential sources of error.

Glossary of Key Terms

TermDefinitionConductorA material that allows the flow of electric current due to the presence of free charge carriers, typically electrons.Drift velocityThe average velocity of charge carriers in a conductor due to an applied electric field. It represents the net movement of electrons superimposed on their random thermal motion.Electric fieldA region of space around a charged object where a force is exerted on other charged objects. The direction of the electric field is the direction of the force on a positive test charge.Conventional currentThe flow of positive charge, historically defined before the discovery of the electron. In metallic conductors, current is actually carried by negatively charged electrons moving in the opposite direction.Potential differenceThe difference in electric potential energy per unit charge between two points in an electric circuit. It is also called voltage.Fermi velocityThe average velocity of electrons in a conductor at room temperature due to their thermal energy. It is typically much higher than drift velocity.ResistanceThe opposition to the flow of electric current in a material. It is measured in ohms (Ω).ElectronA subatomic particle with a negative charge that orbits the nucleus of an atom.AtomThe basic building block of matter, consisting of a nucleus containing protons and neutrons, surrounded by electrons.EJSS modelAn Easy JavaScript Simulations (EJSS) model used to visualize and understand the concept of drift velocity in metallic conductors.

What is drift velocity in conductors?

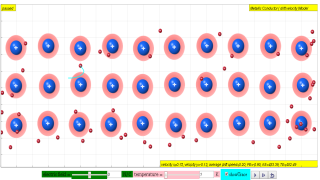
This model has 30 atoms mass=1000 u, charge = +1 unit, and 50 electrons, each 1 u, charge = -1 unit, demonstrates the drift velocity, the flow velocity of in this case negative charge carriers such as electrons, attains due to an electric field. It can also be referred to as axial drift velocity. In general, an electron will 'rattle around' randomly in a conductor at the Fermi velocity. An applied electric field will give this random motion a small net flow velocity in one direction.

When a battery is connected to a wire, the battery will maintain a potential difference +(left) and -(right) for example between the two ends of the wire. In that situation, there will be a field in the wire setup pointing to the right, and charges will flow to the left(and keep flowing) in response to this field.

The mobile charges carriers (often electrons) move about randomly, scattering off atoms and molecules. When there is no electric field in the conductor, there is no net charge flow.

Applying a potential difference, which sets up a field, is much like creating a pressure difference in a gas. In both cases there will be a net flow of charges or gas particles respectively.

For the charge carriers in a metal simulation-model, the applied field when E = positive, it superimposes a net drift velocity (or average speed) vd to the left onto the random motion previously there.

Similarly, when the applied field when E = negative, it superimposes a net drift velocity (or average speed) vd to the right onto the random motion previously there.

How to Use?

1. select from the sliders or enter values via the input fields and click on the “play” button.
2. at electric field E = 0, the 50 electrons (negative charge particles) 'rattle around' randomly in a conductor of 30 atoms as arranged

http://weelookang.blogspot.sg/2015/05/ejss-drift-velocity-model.html EJSS drift velocity model author: Francisco Esquembre , Universidad de Murcia; Maria Jose Cano; lookang http://weelookang.blogspot.sg/

3. notice sometimes when the electrons travel to near each other, they tend to repel as they have the like charges.
4. notice the atoms vibrate in their fixed lattice positions, and with increasing temperature, the vibrations have higher kinetic energies and higher amplitudes.

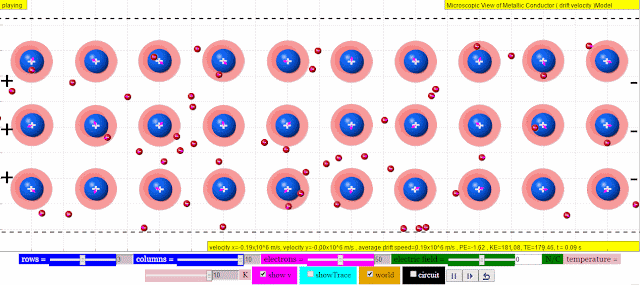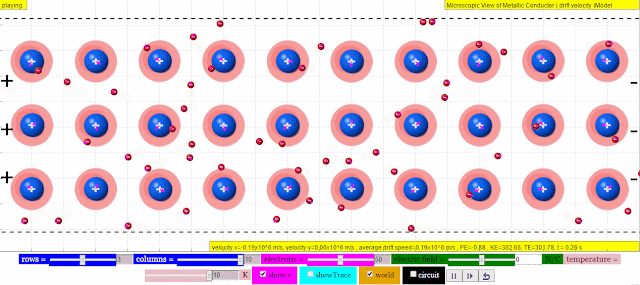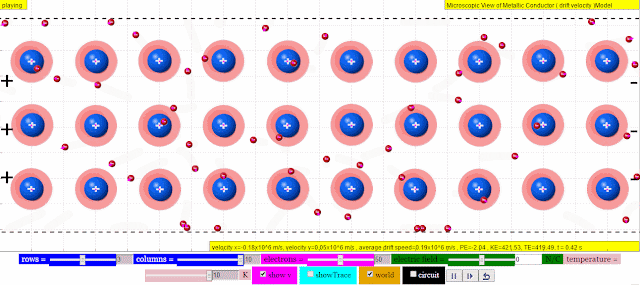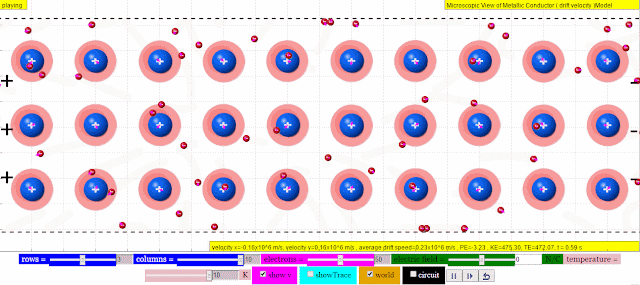http://weelookang.blogspot.sg/2015/05/ejss-drift-velocity-model.html EJSS high temperature drift velocity model author: Francisco Esquembre , Universidad de Murcia; Maria Jose Cano; lookang http://weelookang.blogspot.sg/

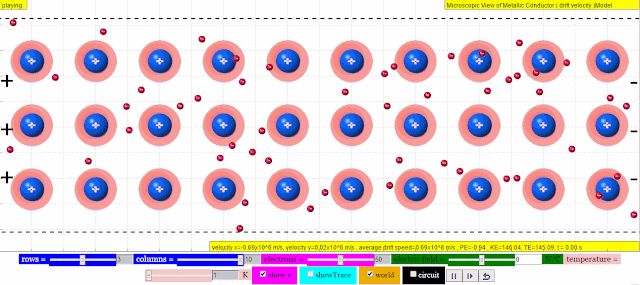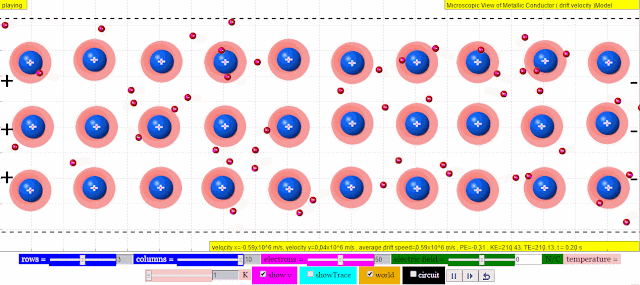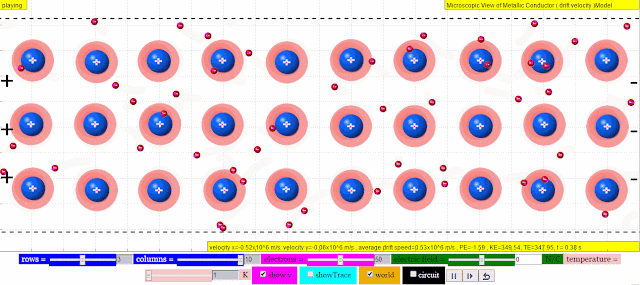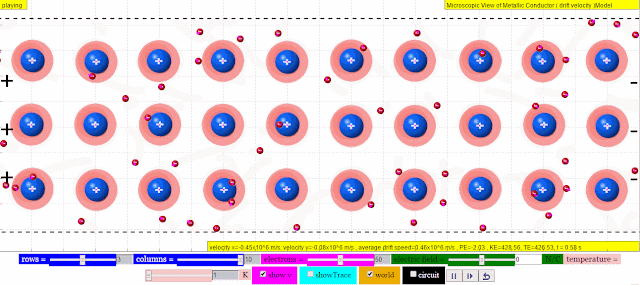
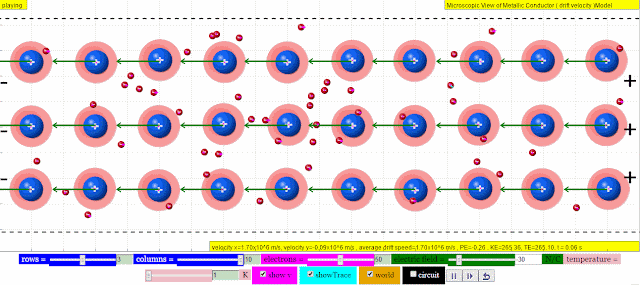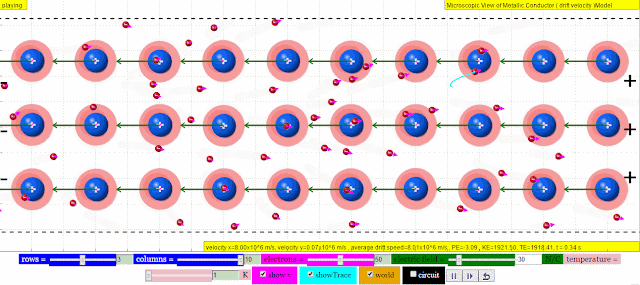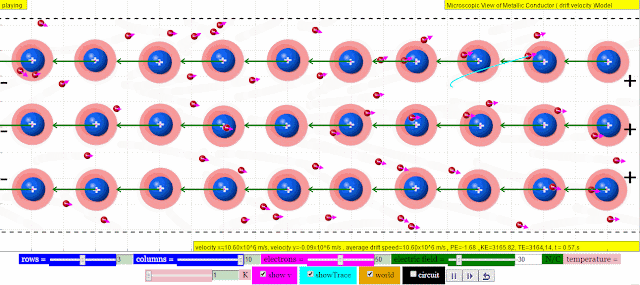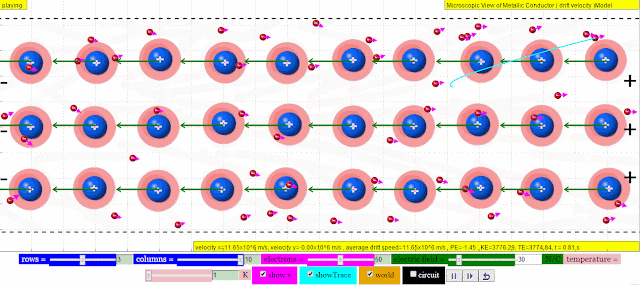
5. Select checkbox "showTrace" will show the path travelled by a single electron to ease your visualization of the path and allow your deduction on the average velocities of the electrons, thus the drift velocity along the direction of the conductor current and opposite to the electric field.

http://weelookang.blogspot.sg/2015/05/ejss-drift-velocity-model.html EJSS positive electric field model with trace author: Francisco Esquembre , Universidad de Murcia; Maria Jose Cano; lookang http://weelookang.blogspot.sg/

 

http://weelookang.blogspot.sg/2015/05/ejss-drift-velocity-model.html EJSS negative electric field model with trace author: Francisco Esquembre , Universidad de Murcia; Maria Jose Cano; lookang http://weelookang.blogspot.sg/

6. Hence or otherwise, describe net drift velocity in metal conductor and explain the factors which affects net drift velocities.

Other interesting fun activities

1. increase the temperature, this model can be used to visualise the effects of increased temperature on the vibrations of atoms fixed in their relative positions like a solid. This can help students figure out that increase in temperature can have an effect on the drift velocity of charge carriers and the resultant opposite direction current flow.

2. Notice potential energy, kinetic energy and total energy are added to allow linking of knowledge to kinetic theory of gases.

3. reversing the electric field while the simulation is playing, the drift velocity allows student to link this idea to the non-instantaneous lighting up of light bulbs when electric circuits are connected, plan and conduct your own experiment to collect evidences to support or rebut this claim.

4. explore your own scenario here to test your initial understanding, thereby showing an appreciation of the atomic structure and mobile charge carriers electrons.

5. How can a lot of loose electrons cause a metal to be a good conductor? This is because each electron is an electric charge that is free to move. Moving electric charges can make up an opposite direction current. But this is only true if they move the same net direction at a drift velocity.

5. leave me a Google+ answer/comment/question/requests etc here http://weelookang.blogspot.sg/2015/05/ejss-drift-velocity-model.html

 Video

 https://notebooklm.google.com/notebook/9ad8b448-f89b-4716-b8ef-4726962afbae/audio

 Version:

 http://weelookang.blogspot.sg/2015/05/ejss-drift-velocity-model.html

https://weelookang.blogspot.com/2024/11/exploring-drift-velocity-and-current-in.html 

Other Resources

[text]

Metallic Conductor and Drift Velocity FAQ

1. What is drift velocity?

Drift velocity is the average velocity that charged particles, like electrons, attain in a material due to an electric field. While these particles have their own random thermal motion, the electric field imposes a directional force, leading to a net movement in one direction.

Imagine a river with a gentle current. Leaves floating on the surface will move randomly due to wind and eddies, but the current gives them a net downstream movement. This downstream movement is analogous to drift velocity.

2. How does drift velocity relate to electric current?

Electric current is the flow of electric charge. In conductors, like metals, the free electrons act as charge carriers. When an electric field is applied (e.g., by connecting a battery), these electrons experience a drift velocity, resulting in a net flow of charge – an electric current. The direction of the current is opposite to the direction of electron flow.

3. Why are metals good conductors of electricity?

Metals have a unique atomic structure where their outermost electrons are loosely bound and free to move throughout the material. These "free electrons" are readily available to constitute an electric current when an electric field is applied, leading to a drift velocity.

4. How does temperature affect drift velocity?

Increased temperature leads to greater thermal vibrations of atoms in a conductor. These vibrations increase the likelihood of collisions between the free electrons and the atoms, hindering their movement. Therefore, drift velocity generally decreases with rising temperature.

5. What is the role of an electric field in creating drift velocity?

An electric field applies a force on charged particles. In a conductor, the electric field created by a potential difference (e.g., from a battery) accelerates the free electrons, giving them a net velocity in a specific direction, hence creating a drift velocity.

6. How can the drift velocity be visualized?

The provided simulation model demonstrates drift velocity by showing the movement of electrons in a conductor. The simulation allows you to manipulate the electric field and temperature to observe their effects on the electrons' movement and visualize the concept of drift velocity.

7. What are some real-world implications of drift velocity?

Drift velocity helps explain phenomena like the non-instantaneous lighting of light bulbs in a circuit. The time delay in illumination is due to the finite drift velocity of electrons in the wires.

8. How can I further explore the concept of drift velocity?

The simulation model provides various tools to investigate the relationship between electric field, temperature, and drift velocity. You can adjust parameters, observe electron behavior, and conduct virtual experiments to deepen your understanding of this fundamental concept in electricity.